Tag: music

Posted on

The Structure of Sound: Network Insights into Bach’s Music

by Ian Scheffler

Representing Bach’s pieces as networks reveals hidden structures in his music. (Credit: Suman Kulkarni)

Even today, centuries after he lived, Johann Sebastian Bach remains one of the world’s most popular composers. On Spotify, close to seven million people stream his music per month, and his listener count is higher than that of Mozart and even Beethoven. The Prélude to his Cello Suite No. 1 in G Major has been listened to hundreds of millions of times.

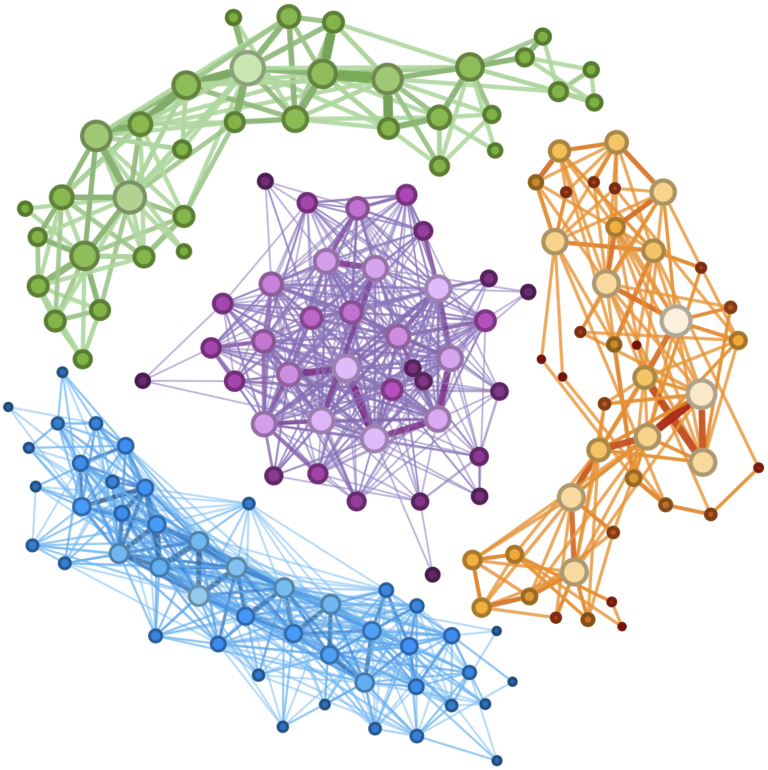
What makes Bach’s music so enduring? Music critics might point to his innovative harmonies, complex use of counterpoint and symmetrical compositions. Represent Bach’s music as a network, however, where each node stands for one musical note, and each edge the transition from one note to another, and a wholly different picture emerges.

In a recent paper in Physical Review Research, Dani S. Bassett, J. Peter Skirkanich Professor in Bioengineering and in Electrical and Systems Engineering within the School of Engineering and Applied Science, in Physics & Astronomy within the School of Arts & Sciences, and in Neurology and Psychiatry within the Perelman School of Medicine, and Suman Kulkarni, a doctoral student in Physics & Astronomy, applied network theory to Bach’s entire oeuvre.

The paper sheds new light on the unique qualities of Bach’s music and demonstrates the potential for analyzing music through the lens of networks. Such analysis could yield benefits for music therapists, musicians, composers and music producers, by giving them unprecedented quantitative insight into the structure of different musical compositions.

“This paper provides a starting point for how one can boil down these complexities in music and start with a simple representation to dig into how these pieces are structured,” says Kulkarni, the paper’s lead author. “We applied this framework to a dozen types of Bach’s compositions and were able to observe quantitative differences in how they were structured.”

Read the full story in Penn Engineering Today.

Posted on

How to Learn About a World-class Double Bass? Give it a CT

by Darcy Lewis  

The instrument imaging team, from left: Philadelphia Orchestra bassist Duane Rosengard; Peter Noël, PhD, director of CT Research at the Perelman School of Medicine; luthier Zachary S. Martin; Leening Liu, a PhD student in Noël’s Laboratory of Advanced Computed Tomography Imaging; and Mark Kindig.

When you’re an expert in medical CT imaging, two things are bound to happen, says Peter Noël, PhD, associate professor of Radiology and director of CT Research at the Perelman School of Medicine. One: You develop an insatiable curiosity about the inner workings of all kinds of objects, including those unrelated to your research. And two: Both colleagues and complete strangers will ask for your help in imaging a wide variety of unexpected items.

Over the course of his career, in between managing his own research projects, Noël has imaged diverse objects ranging from animal skulls to tree samples from a German forest, all in the name of furthering scientific knowledge. But none has intrigued him as much as his current extracurricular project: the first known attempt to perform CT imaging of some of the world’s finest string basses. 

The goal is to crack the code on what makes a world-class instrument. This knowledge could both increase the ability to better care for masterworks built between the 17th and 19th centuries, as well as providing insights into refining the building of new ones, including possibly shifting from older, scarcer European wood to the use of sustainably harvested U.S. wood.

That’s why Noël and Leening Liu, a PhD student in Noël’s Laboratory of Advanced Computed Tomography Imaging, have found themselves volunteering to run the basses through a Penn CT scanner occasionally, when they’re not developing next-generation CT technology. 

“We always learn something out of projects like this … the more appealing part is that medical research can also be applied to non-medical things,” Noël said. “We have the opportunity to take what we learn in medicine and use it for something else—in this case, moving the arts forward.”

Read the full story in Penn Medicine News.

Peter Noël is Assistant Professor of Radiology in the Perelman School of Medicine and member of the Penn Bioengineering Graduate Group.

Leening Liu is a Ph.D. student in Bioengineering. She is a member of the Laboratory for Advanced Tomography Imaging (LACTI) with research interests including clinical applications of spectral CT and spectral CT thermometry.

Posted on

Bioengineering and BDM Go Together

by Joe Maggiore, Bioengineering ’19

BDM stage
Band Dance Music in performance

I’m a rising junior studying Bioengineering at Penn. I’m also the founder of a music group called Band Dance Music (BDM). The overall premise of the group is to take the same music that a DJ plays at a college party but to play it with an 11-piece live band. The idea for this group started before I got to Penn, but it was something that I was confident in pursuing despite all of the other time commitments during the school year.

Starting a band at Penn was definitely a challenge. There are already so many music groups on Penn’s campus that it’s very easy for a group that is just starting out to get drowned out by other more prominent groups. After really pushing marketing hard for auditions, it actually was pretty easy to find students who were interested in the idea behind the group. Interestingly, of the 11 members that are now in the group, nine of them are actually in the School of Engineering and Applied Science.

While bioengineering and band dance music seem like two totally disparate fields, I was actually able to bridge the gap between these areas while taking ENGR105 with Professor Rizk. At the end of this course, we are asked to create a graphical user interface (GUI) that combines the entire course’s material. This GUI is completely free form – it can be in any area of interest that you like.

Since for a while I’d been having trouble arranging music completely by ear, I thought this would be the perfect opportunity to create a GUI that would help me arrange music for the band. There is rarely free time to spare during the school year, so being able to work on a passionate project of mine while also being able to complete my course work was a win-win situation. The GUI definitely took me longer than expected to create since it involved having to process electronic music into parts that would be easier to arrange, but I eventually was able to finish the interface. It featured a tap metronome, a filtering system, and a visual music player so I could streamline the music writing process. Below is a pictures of the GUI I created.

BDM is always looking for more interesting people to join who have a passion for this unique concept for a band. If any bioengineers reading out there are interested, feel free to reach out to me – I’d love to talk more about it. Thanks for reading!